Question

What is the area of wxyz with vertices w(0,1), x(3,9), y(-1,8), z(-4,5) to the nearest unit

Answer 1

do you have a picture?

thanks

Answer 2

If you typed in the coordinates correctly, then there is only ONE 90° angle. So you will have to draw line segment WY to create 2 triangles. Use the distance formula to find the lengths of the sides. Use the right triangle area formula for ΔWYZ and use Heron's formula for Area of ΔWYX and add the two triangles together to get the area of the quadrilateral.

ΔWYZ

WZ = 4√2

ZY = 3√2

A =
`(1)/(2)`* 4√2 * 3√2

=
`(1)/(2)` * 4 * 3 * 2

= 4 * 3

= 12

ΔWYX

WY = 5√2 ≈ 7.1

XY = √17 ≈ 4.1

WX = √73 ≈ 8.5

A =
`√(19.7(19.7 - 7.1) + 19.7(19.7 - 4.1) + 19.7(19.7-8.5))`

=
`√(19.7(12.6) + 19.7(15.6) + 19.7(11.2))`

=
`√(242.22 + 307.32 + 220.64)`

=
`√(790.18)`

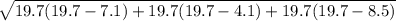
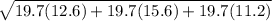
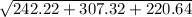
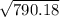
= 28.11

ΔWYZ + ΔWYX

12 + 28.11

= 40.11

Answer: 40.11 units²