Question

What is the measure of RST shown in the diagram below

Answer 1

Answer is 64

Explanation:

Answer 2

A.
`m\angle RST=64^o`

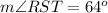
Explanation:

We can see from our given diagram that angle RST is formed by intersection of two tangents of our circle.

We will use Tangent-Tangent Angle theorem, which states that if an angle is formed by two intersecting tangents, then the measure of the angle is one-half the difference of the measures of the intercepted arcs (the major arc - the minor arc).

`m\angle RST=(1)/(2)(\text{Major arc- Minor arc})`

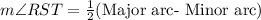
We can see that major arc is RT and minor arc is UV.

Upon substituting measures of our major arc and minor arc we will get,

`m\angle RST=(1)/(2)(153^o-25^o)`

`m\angle RST=(1)/(2)(128^o)`

`m\angle RST=64^o`

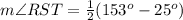
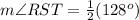
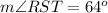
Therefore, measure of RST is 64 degrees and option A is the correct choice.