Question

Simplify the expression given below

Answer 1

A.
`(1)/((r+1)\cdot (r-2))`

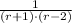
Explanation:

We have been given an expression
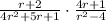`(r+2)/(4r^2+5r+1)\cdot (4r+1)/(r^2-4)`. We are asked to simplify our given expression.

We will factor the denominators of both fractions as shown below:

`4r^2+5r+1`

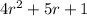
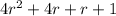`4r^2+4r+r+1` Splitting the middle term.

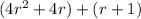`(4r^2+4r)+(r+1)` Making groups.

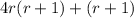`4r(r+1)+(r+1)` Factor out 4r from 1st group.

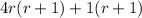`4r(r+1)+1(r+1)` Factor out 1 from 2nd group.

`(4r+1)(r+1)`

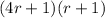
Using difference of squares we will factor the 2nd denominator as:

`r^2-4=r^2-2^2=(r+2)(r-2)`

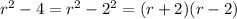
Substituting these values in our given problem we will get,

`(r+2)/((4r+1)(r+1))\cdot (4r+1)/((r+2)(r-2))`

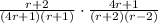
After cancelling out terms we will get,

`(1)/((r+1))\cdot (1)/((r-2))`

`(1)/((r+1)\cdot (r-2))`

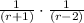
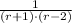
Therefore, option A is the correct choice.

Answer 2

The correct option is A.

Explanation:

The given expression is

`(x+2)/(4x^2+5x+1)* (4x+1)/(x^2-4)`

`(x+2)/(4x^2+4x+x+1)* (4x+1)/(x^2-2^2)`

`(x+2)/(4x(x+1)+1(x+1))* (4x+1)/((x-2)(x+2))`

`(x+2)/((x+1)(4x+1))* (4x+1)/((x-2)(x+2))`

`((x+2)(4x+1))/((x+1)(4x+1)(x-2)(x+2))`

Cancel out common factors.

`(1)/((x+1)(x-2))`

Therefore option A is correct.