Question

PLEASE HELP Given: △KLM LM=12, m∠K=60°, m∠M=45° Find: Perimeter of △KLM.

Answer 1

35.2 units.

Explanation:

We have been given that in triangle KLM, side LM is 12 units and masure of angle K is 60 degrees and measure of angle M is 45 degrees.

We will use law of sines to find the length of side MK and KL as:

`\frac{a}{\text{sin}(A)}=\frac{b}{\text{sin}(B)}=\frac{c}{\text{sin}(C)}`

`\frac{KL}{\text{sin}(45^(\circ))}=\frac{12}{\text{sin}(60^(\circ))}`

`\frac{KL}{\text{sin}(45^(\circ))}*\text{sin}(45^(\circ))=\frac{12}{\text{sin}(60^(\circ))}*\text{sin}(45^(\circ))`

`KL=(12)/(0.866025403784)*0.707106781187`

`KL=13.85640646*0.707106781187`

`KL=9.79795897\approx 9.8`

`\frac{MK}{\text{sin}(75^(\circ))}=\frac{12}{\text{sin}(60^(\circ))}`

`\frac{MK}{\text{sin}(75^(\circ))}*\text{sin}(75^(\circ))=\frac{12}{\text{sin}(60^(\circ))}*\text{sin}(75^(\circ))`

`MK=(12)/(0.866025403784)*0.965925826289`

`MK=13.85640646*0.965925826289`

`MK=13.38426\approx 13.4`

`\text{Perimeter of triangle KLM}=LM+MK+KL`

`\text{Perimeter of triangle KLM}=12+13.4+9.8`

`\text{Perimeter of triangle KLM}=35.2`

Therefore, perimeter of triangle KLM is 35.2 units.

Answer 2

We have to find the perimeter of the triangle KLM.

We have been given that the length of the side LM=12,
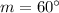`m\angleK=60^\circ`, and
`m\angle M= 45^\circ`

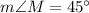
Refer the attached image.

In a triangle sum of three angles should be
`180^\circ`.

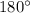So,

`m\angle K+m\angle L+m\angle M=180^\circ`

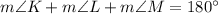
Plugging the values of angle K and angle M, we get:

`60^\circ+m\angle L+45^\circ=180^\circ`

So,

`m\angle L=180^\circ-105^\circ=75^\circ`

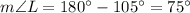
Now, that we have the measure of angle L, we will apply sine rule to find the length of the sides KL and KM.

Using the sine law for the triangle KLM, we get:

`(sin K)/(LM)=(sin L)/(KM)=(sin M)/(KL)`

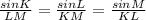
Refer the image. Plugging the value of the sides of the triangle KLM and the angles of the triangle KLM, we get:

`\frac {sin 60^\circ}{12}=(sin 75^\circ)/(y)=(sin 45^\circ)/(x)`

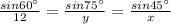
Now using,

`\frac {sin 60^\circ}{12}=(sin 75^\circ)/(y)`

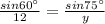
We get the value of 'y'

`y=(sin 75^\circ)/(sin 60^\circ) * 12=(0.9659)/(0.866) * 12=13.38`

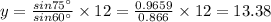
So the length of the side KM is 13.38 units.

Now using,

`\frac {sin 60^\circ}{12}=(sin 45^\circ)/(x)`

We get the value of 'x'

`x=(sin 45^\circ)/(sin 60^\circ) * 12=(0.707)/(0.866) * 12=9.79`

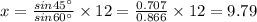
So the length of the side KL is 9.79 units.

Now, to find the perimeter of the triangle KLM we need to sum up the length of the sides of the triangle KLM.

The perimeter of the triangle KLM
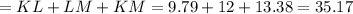`= KL+ LM + KM = 9.79 + 12 + 13.38 = 35.17` units