Question

The equilibrium constant for the equilibrium, 3A+ 2B ↔ 2D + E, is 4.22 x 10-3 . What is the equilibrium constant for the equilibrium: D + (1/2)E ↔ (3/2)A + B?

2.1110-3

237

-2.1110-3

15.4

Answer 1

Option D: 15.4

There are following rules for manipulating equilibrium constant:

1. On adding two equilibrium reactions, their equilibrium constant gets multiplied.

For example:
`A\rightarrow B (K_(1))`

`C\rightarrow D(K_(2))`

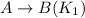
On adding two reactions,

`A+C\rightarrow B+D(K=K_(1)* K_(2))`

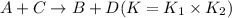
2. On subtracting two equilibrium reactions, their equilibrium constant gets divided.

For example:
`A\rightarrow B (K_(1))`

`C\rightarrow D(K_(2))`

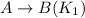
On subtracting two reactions,

`A-C\rightarrow B-D`

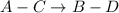
Or,

`A+D\rightarrow B+C(K=(K_(1))/(K_(2)))`

3. If an equilibrium reaction is multiplied by any constant, it goes to the power of its equilibrium constant.

For example:
`A\rightarrow B (K_(1))`

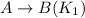
Thus,

`2A\rightarrow 2B (K=K_(1)^(2))`

4. On reversing an equilibrium reaction, the equilibrium constant of reversed reaction becomes inverse of the original value.

For example:
`A\rightarrow B (K_(1))`

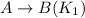
Thus,

`B\rightarrow A (K=(1)/(K_(1)))`

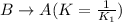
Now, the given equilibrium reaction is as follows:

`3A+2B\rightleftharpoons 2D+E (K=4.22* 10^(-3))`

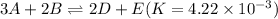
To get the desired reaction, first reverse the above reaction as follows:

`2D+E\rightleftharpoons 3A+2B\left ( K=(1)/(4.22* 10^(-3)) \right )`

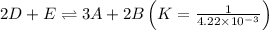
Now, multiply the above reaction with 1/2,

`D+1/2E\rightleftharpoons 3/2A+B\left ( K=\left ((1)/(4.22* 10^(-3))  \right )^(1/2) \right )`

Thus,

`K=\left ((1)/(4.22* 10^(-3)) \right )^(1/2)=15.4`

Therefore, equilibrium constant for the resultant reaction is 15.4 that is option D.