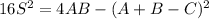Question

Three points lie on the same straight line. If each point can act as an endpoint, how many distinct line segments can be formed using the set of points?

A. one

B. three

C. four

D. five

E. two

Answer 1

It's like a collinear triangle, we get three sides, one between each pair.

Answer: B

The Pythagorean Theorem is
`a^2+b^2=c^2` where a,b, and c are the sides of a right triangle. There's a dual called Archimedes Theorem relating the three squared areas A,B,C formed from three collinear points:

`\pm √(A) \pm √(B) = \pm √(C)`

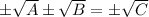
which is better expressed as

`0 = (A+B+C)^2-2(A^2+B^2+C^2)`

I wrote it this way to mention that when A,B,C are the squared sides of a triangle, i.e. from three non-collinear points, this quantity is the sixteen times the squared area of the triangle.

Also when this quantity is negative, it means we have three squared lengths which don't satisfy the triangle inequality.

Your teacher will probably never teach you this secret formula for the area of a triange S given the three squared sides A,B,C:

`16S^2 =(A+B+C)^2-2(A^2+B^2+C^2)`

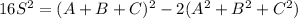
An equivalent, more useful version is

`16S^2 = 4AB-(A+B-C)^2`