Question

A golfer gives a ball a maximum initial speed of 51.5 m/s. how far does it go

Answer 1

Golf ball will go a maximum of 270.36 meter.

Step-by-step explanation:

Projectile motion has two types of motion Horizontal and Vertical motion.

Vertical motion:

We have equation of motion, v = u + at, where v is the final velocity, u is the initial velocity, a is the acceleration and t is the time taken.

Considering upward vertical motion of projectile.

In this case, Initial velocity = vertical component of velocity = u sin θ, acceleration = acceleration due to gravity = -g
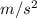`m/s^2` and final velocity = 0 m/s.

0 = u sin θ - gt

t = u sin θ/g

Total time for vertical motion is two times time taken for upward vertical motion of projectile.

So total travel time of projectile = 2u sin θ/g

Horizontal motion:

We have equation of motion ,
`s= ut+(1)/(2) at^2`, s is the displacement, u is the initial velocity, a is the acceleration and t is the time.

In this case Initial velocity = horizontal component of velocity = u cos θ, acceleration = 0
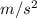`m/s^2` and time taken = 2u sin θ /g

So range of projectile,
`R=ucos\theta*(2u sin\theta)/(g) = (u^2sin2\theta)/(g)`

Now in the given problem

A golfer gives a ball a maximum initial speed of 51.5 m/s. how far does it go

u = 51.5 m/s, for maximum range θ = 45⁰

So maximum distance reached =
`(51.5^2sin(2*45))/(9.81)=270.36 meter`

So it will go a maximum of 270.36 meter.