Question

Help!!! What is the difference ? (x - 8)/(3x) - (3x)/(x ^ 2)

Answer 1

Given that you're providing only a single term, asking for a difference doesn't make a lot of sense. I assume you're asking to have the expression simplified?

If so, then it can be reduced to (x - 17) / 3x

Explanation:

You can simplify by multiplying both terms each by a different ratio that equals one, but also gives the terms common denominator. Multiplying the first one by x²/x² and the second by 3x/3x will do that for you.

After that you can group the terms into one fraction and simplify:

`(x - 8)/(3x) - (3x)/(x^2) \\\\= (x - 8)/(3x)*(x^2)/(x^2) - (3x)/(x^2) *(3x)/(3x) \\\\= (x^3 - 8x^2)/(3x^3) - (9x^2)/(3x^3) \\\\= (x^3 - 8x^2 - 9x^2)/(3x^3) \\\\= (x - 8 - 9)/(3x)\\\\= (x - 17)/(3x)\\`

Answer 2

The difference of
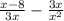`(x-8)/(3x)-(3x)/(x^2)` is
`\mathbf{(x-17)/(3x)}`

Explanation:

We need to find the difference:
`(x-8)/(3x)-(3x)/(x^2)`

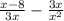
Simplifying to find the difference:

`(x-8)/(3x)-(3x)/(x^2)`

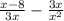
x is common in both 3x and x^2 so, we can cancel it out and we get:

`=(x-8)/(3x)-(3x)/(x(x))\\=(x-8)/(3x)-(3)/(x)`

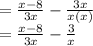
Now, taking LCM of 3x and x we get 3x, We will multiply 3x with both terms and then solve we get:

`=(x-8-3(3))/(3x)\\=(x-8-9)/(3x)\\=(x-17)/(3x)`

It cannot be further simplified, so we get
`(x-17)/(3x)` as answer.

So, The difference of
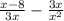`(x-8)/(3x)-(3x)/(x^2)` is
`\mathbf{(x-17)/(3x)}`