Question

MATH HELP!!!!!!!!!!!!!!!

Answer 1

If you don't want to compare infinite decimal places, you can simply convert 0.3 to fraction instead of 1/3 to number:

`0.3 = (3)/(10)`

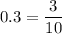
So, the question now becomes: which is bigger, 1/3 or 3/10?

You can find the answer by giving the same denominator to both fractions:

`(3)/(10) = (3\cdot3)/(10\cdot 3) = (9)/(30)`

`(1)/(3) = (1\cdot 10)/(3 \cdot 10) = (10)/(30)`

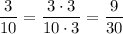
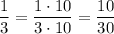
So, since 10/30 is more than 9/30, you can deduce that 1/3 is more than 0.3

Answer 2

1/3 because it is 0.333333 repeated while 0.3 is just 0.30000 so just covert them to decimals