Question

Two hikers are 77 miles apart and walking toward each other. they meet in 10 hours. find the rate of each hiker if one hiker walks 3.3 mph faster than the other.

Answer 1

The rate of the slower hiker is 2.2 mph and the rate of the faster hiker is 5.5 mph

Step-by-step explanation

One hiker walks 3.3 mph faster than the other.

Suppose, the speed of the slower hiker is
`x` mph.

So, the speed of the faster hiker will be:
`(x+3.3) mph`

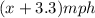
Both hikers are walking toward each other and meet in 10 hours.

We know that,
`Distance= speed*time`

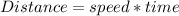
So, the distance walked by the slower hiker in 10 hours
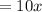`=10x` miles and the distance walked by the faster hiker in 10 hours
`= 10(x+3.3)` miles.

Given that, they were 77 miles apart in the beginning. So the equation will be ...

`10x+10(x+3.3)=77\\ \\ 10x+10x+33=77\\ \\ 20x=77-33= 44\\ \\ x= (44)/(20)=2.2`

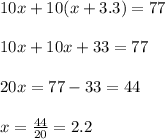
Thus, the rate of the slower hiker is 2.2 mph and the rate of the faster hiker is (2.2 + 3.3) mph = 5.5 mph