Area of rhombus =
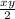
where x and y are diagonals.
Given length of one diagonal is 4.5 dm
So, let x= 4.5dm
1 dm = 10 cm
4.5dm= 45cm
area =
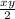
so,
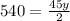
y = 24 cm
The two diagonals are x= 45cm and y = 24cm
Since diagonals bisect each other, we get 22.5cm and 12cm
Using right triangle formula
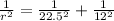
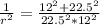
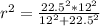
r = 10.588cm
Distance of center to the side =
 = 5.294 cm
= 5.294 cm