Question

Graph the lines by finding the points of intersection with the axes (intercepts):

y=− 1/2 x−1

Answer 1

Graph in attachment.

Explanation:

Given:
`y=-(1)/(2)x-1`

To graph the line using intercepts with axis.

x-intercept and y-intercept

• For x-intercept, Put y=0 into equation

`0=-(1)/(2)x-1`

`x=-2`

x-intercept: (-2,0)

• For y-intercept, Put x=0 into equation

`y=-(1)/(2)\cdot 0-1`

`y=-1`

y-intercept: (0,-1)

Now draw the graph by plotting the points.

Please have a look with attachment for graph.

Answer 2

To solve this problem you must apply the proccedure shown below:

1. You have the following function:

`y=-(1)/(2) (x-1)`

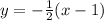
2. You can rewrite it as following:

`y=((-x+1))/(2)`

3. The line intersects the y-axis when
`x=0`, therefore:

`y=((0+1))/(2)=(1)/(2) =0.5`

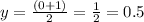
4. The line intersects the x-axis when
`y=0`:

`0=((-x+1))/(2)\\ 0=-x+1\\ x=1`

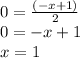
5. Now plot the points (0, 0.5) and (1,0) in a graph , as you can see in the figure attached.

Therefore, the answer is: The points of intersection are (0, 0.5) and (1,0).