Question

*27 POINTS PLEASE HELP*

The coordinates of the vertices of quadrilateral ABCD are A(-4,-1) B(-1,2) C(5,1) and D(1,-3)

Drag and drop the choices into each box to correctly complete the sentences

Answer 1

To compute the slope of a segment, given its endpoints
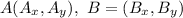`A(A_x,A_y),\ B=(B_x,B_y)`, you write

`m_(AB) = (A_y-B_y)/(A_x-B_x)`

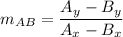
Given this formula, you have the four slopes:

`m_(AB) = (A_y-B_y)/(A_x-B_x) = (-1-2)/(-4+1) = (-3)/(-3) = 1`

`m_(BC) = (B_y-C_y)/(B_x-C_x) = (2-1)/(-1-5) = (1)/(-6) = (-1)/(6)`

`m_(CD) = (C_y-D_y)/(C_x-D_x) = (1+3)/(5-1) = (4)/(4) = 1`

`m_(DA) = (D_y-A_y)/(D_x-A_x) = (-3+1)/(1+4) = (-2)/(5) = -(2)/(5)`

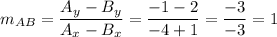
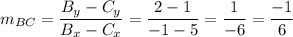
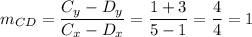
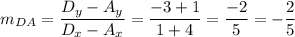
Now, two segments are parallel if they have the same slope. So, AB and CD are parallel, because they both have slope 1, but BC and DA are not parallel.

This means that ABCD is not a parallelogram, because in parallelograms the opposite sides are parallel.