Notice that the pattern is "previous term plus 2". This is an arithmetic sequence where the difference (d) equals +2
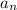 = a₁ + d(n - 1) ; where a₁ is the first term, d is the difference, and n is the term.
= a₁ + d(n - 1) ; where a₁ is the first term, d is the difference, and n is the term.
f(n) = 1 + 2(n - 1)
f(n) = 1 + 2n - 2
f(n) = 2n - 1
********************************
f(10) = 2(10) - 1
f(10) = 20 - 1
f(10) = 19