2 Answers

Aet · Answer 1 · 2019-09-06T14:20:59+0000

We have been given a piece wise function in which one graph is of parabola and other one is of a straight line.

When we shift the graph of
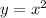y=x^2 above by 6 units then the equation of the graph is

y=x^2+6 .

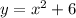Since, the given graph of the parabola full fills this criteria. Hence, the equation of the parabola is given by

y=x^2+6

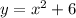
The graph of the parabola is up to x=3. At point x=3 there is an open circle. Hence, we must include less than sign.

Now, the equation of the line is given by
y=-x+6

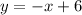
We have a solid sign , hence we must include greater than equal to sign.

Therefore, the equation for the piece wise function is given by

$y=\left\{\begin{matrix} x^2=6, x<3\\  \\ -x+6, x\geq 3  \end{matrix}\right.$

C is the correct option.

Please log in or register to add a comment.