Question

H(x)= (x^2 -36)/x +6 explain why the following function is not continuous at x=-6

Answer 1

You can solve this problem through factoring.

First, you have the equation,

`h(x) = (x^2-36)/(x-6)`

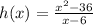
Then, you can factor the numerator.

`h(x) = ((x+6)(x-6))/(x-6)`

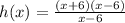
You can cancel out the x-6 in both the numerator and the denominator because they would equal to just 1.

You are left with
`h(x) = x+6`

The function is removable noncontinuous at x=6 because if you plug in 6 in x-6, your denominator would be undefined.