Question

Assume that there are 22 frozen dinners: 12 pasta, 6 chicken, and 4 seafood dinners. The student selects 5 of them. What is the probability that at least 2 of the dinners selected are pasta dinners?

Answer 1

Since, there are 22 frozen dinners. Out of these, 22 are pasta, 6 chicken and 4 seafood dinners.

We have to determine the probability that at least 2 of the dinners selected are pasta dinner. At least 2 of the dinners selected are pasta, it means that there can be 2 pasta, 3 , 4 or 5.

So, Probability with at least 2 of dinners

=
`((^(12)C_(2) * ^(10)C_(3))+ (^(12)C_(3) * ^(10)C_(2))+(^(12)C_(4) * ^(10)C_(1))+(^(12)C_(5)))/(^(22)C_(5))`

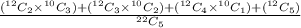
We will use the combination formula, which states
`^nC_r = (n!)/(r! (n-r)!)`

=
`((66 * 120)+(220 * 45)+(495 * 10)+(792))/(26334)`

=
`(7920+9900+4950+792)/(26334)`

=
`(23562)/(26334)`

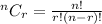
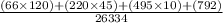
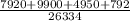
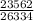
= 0.894

=0.90

Therefore, the probability that at least 2 of the dinners selected are pasta is 0.90