To answer this question it must be taken into account that according to the kinematics equations for the position of an object that performs this type of movement is:
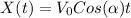
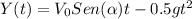
Where:
X (t) is the position of the object in the horizontal direction as a function of time
Y (t) is the position of the object in the vertical direction as a function of time
α is the angle of inclination in which the object is thrown
 is the initial velocity of the object
is the initial velocity of the object
g is the acceleration of gravity
t is the time
We want to know what distance the projectile traveled in 2.22 seconds after launch.
Then it is:
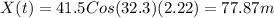
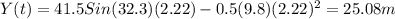
After 2.22 seconds, the projectile strikes with the target at a horizontal distance of 77.87 m and a vertical distance of 25.08 m.