Question

Jose has $6.70 in an assortment of coins. If he has 3 more quarters than pennies,11 more dimes than pennies, and 2.5 times as many nickels as pennies, how many of each type of coin does he have?

Thanks in advance.

Answer 1

He has 10 pennies, 13 quarters , 21 dimes and 25 nickels.

Step-by-step explanation

Suppose, the number of pennies is
`x`

As he has 3 more quarters than pennies, 11 more dimes than pennies and 2.5 times as many nickels as pennies....

So, the number of quarters
`= x+3`,

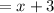the number of dimes
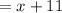`= x+11` and

the number of nickels
`=2.5x`

We know, the value of each penny is $0.01 , value of each quarter is $0.25 , value of each dime is $0.10 and value of each nickel is $0.05

Given that, the total value of all coins is $6.70

So the equation will be.....

`0.01x+0.25(x+3)+0.10(x+11)+0.05(2.5x)=6.70\\ \\ 0.01x+0.25x+0.75+0.10x+1.10+0.125x=6.70\\ \\ 0.485x+1.85=6.70\\ \\ 0.485x=6.70-1.85=4.85\\ \\ x=(4.85)/(0.485)=10`

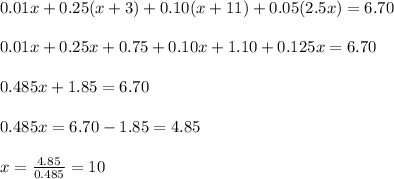
So, the number of pennies is 10 , number of quarters is (10+3)= 13 , number of dimes is (10+11)= 21 and number of nickels is (2.5×10)= 25