The height of the rocket above the ground after
 seconds is given by the equation :
seconds is given by the equation :
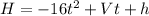 , where
, where
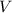 is the initial velocity and
is the initial velocity and
 is the initial height.
is the initial height.
Given that,
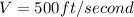 and
and
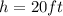
So, the equation will become:

A) For finding the height of the rocket 3 seconds after the launch, we will plug
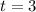 into the above equation. So....
into the above equation. So....
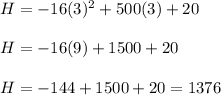
So, the height of the rocket 3 seconds after the launch is 1376 feet.
B) When the rocket at a height of 400 feet, then we will plug
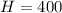
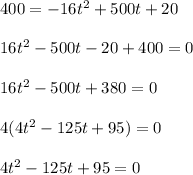
Using quadratic formula, we will get......
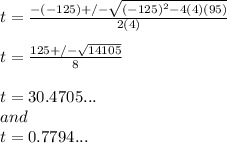
So, after 0.7794...seconds and 30.4705...seconds the rocket is at a height of 400 feet above the ground.
C) The time duration that the rocket remains in the air means we need to find the time taken by the rocket to reach the ground. When it reaches the ground, then
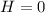 . So.....
. So.....
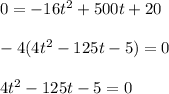
Using quadratic formula, we will get.....

(Negative value is ignored as time can't be in negative)
So, the rocket will remain in the air for 31.2899... seconds.