Question

In a right triangle ABC, CD is an altitude, such that AD=BC. Find AC, if AB=3 cm, and CD= 2 cm.

Answer 1

it is sqrt(7)

Explanation:

when the altitude hits the triangles edge at D, it splits AB in to 2 segments which are ad and db. ad = (3-x) and db =x. and we have 2 right triangles. DCB and ACD are right triangles. so-> looking at BCD (3-x)^2+sqrt(3)^2=x^2, using pythagorean thereom, we get x=2. so then we look and triangle ADC and we see 2^2 +sqrt(3)^2 =7. we take the square root of that to get ac which is sqrt(7)

Answer 2

Consider right triangle ΔABC with legs AC and BC and hypotenuse AB. Draw the altitude CD.

1. Theorem: The length of each leg of a right triangle is the geometric mean of the length of the hypotenuse and the length of the segment of the hypotenuse adjacent to that leg.

According to this theorem,

`BC^2=BD\cdot AB.`

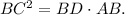
Let BC=x cm, then AD=BC=x cm and BD=AB-AD=3-x cm. Then

`x^2=(3-x)\cdot 3,\\ \\x^2=9-3x,\\ \\x^2+3x-9=0,\\ \\D=3^2-4\cdot (-9)=9+36=45,\\ \\√(D)=√(45)=3√(5),\\ \\x_1=(-3-3√(5) )/(2)<0,\ x_2=(-3+3√(5) )/(2)>0.`

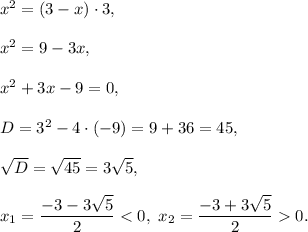
Take positive value x. You get

`AD=BC=(-3+3√(5) )/(2)\ cm.`

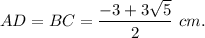
2. According to the previous theorem,

`AC^2=AD\cdot AB.`

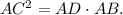
Then

`AC^2=(-3+3√(5) )/(2)\cdot 3=(-9+9√(5) )/(2),\\ \\AC=\sqrt{(-9+9√(5) )/(2)}\ cm.`

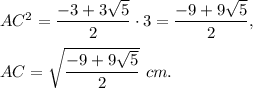
Answer:
`AC=\sqrt{(-9+9√(5) )/(2)}\ cm.`

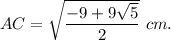
This solution doesn't need CD=2 cm. Note that if AB=3cm and CD=2cm, then

`CD^2=AD\cdot DB,\\ \\2^2=AD\cdot (3-AD),\\ \\AD^2-3AD+4=0,\\ \\D<0`

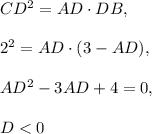
This means that you cannot find solutions of this equation. Then CD≠2 cm.