Question

Can anyone please help me solve this?

Answer 1

Squares everything and then it all work

Answer 2

A)

The Pythagorean theorem states that the sum of the squared legs is the squared hypothenuse:

`a^2+b^2=c^2`

If we divide the whole expression by
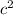`c^2`, we have

`(a^2)/(c^2) + (b^2)/(c^2) = (c^2)/(c^2)`

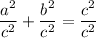
B)

The sine of x is the ratio between the opposite leg and the hypothenuse, while the cosine of x is the ratio between the adjacent leg and the hypothenues:

`\sin(x) = (a)/(c),\quad \cos(x) = (b)/(c)`

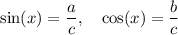
This means that

`\sin^2(x) = \left((a)/(c)\right)^2 = (a^2)/(c^2),\quad \cos^2(x) = \left((b)/(c)\right)^2 = (b^2)/(c^2)`

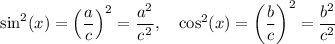
C)

From part B, we know that

`\sin^2(x)+\cos^2(x) = (a^2)/(c^2) + (b^2)/(c^2)`

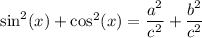
From part A, we know that this sum equals

`\sin^2(x)+\cos^2(x) = (a^2)/(c^2) + (b^2)/(c^2)=(c^2)/(c^2)=1`

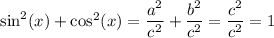
Which terminates the proof.