Question

What is the inverse of f(x)=log(x)

Answer 1

The inverse function of the logarithm is the exponential function:

`f(x) =\log(x) \implies f^(-1)(x) = e^x`

In fact, the expression
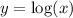`y = \log(x)` means that if you want to obtain x, you have to give y as exponent to e:
`e^y = x`

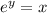
So, we can check both expressions:

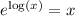`e^(\log(x)) = x`, because this expression means "I am giving to e the following exponent: a number that, when given as exponent to e, gives x".

On the other hand, you have

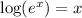`\log(e^x) = x`, because this expression means "what exponent do I have to give to e to obtain e^x?". Well, you've basically already written it: if you want to obtain e^x, you have to give the exponent x.

So, we've shown that
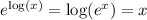`e^(\log(x)) = \log(e^x) = x`, which proves that
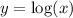`y=\log(x)` and
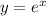`y = e^x` are one the inverse function of the other.