Answer:
Option B. These customers use at least 4,970 gallons but no more than 8,970 gallons of water per month
Explanation:
Let
x -----> the number of gallons of water, used by customers
we know that
The system of inequalities that represent this situation is
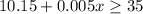 -----> inequality A
-----> inequality A
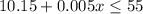 -----> inequality B
-----> inequality B
Solve the inequality A
subtract 10.15 both sides
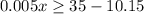
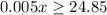
divide by 0.005 both sides
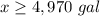
Solve the inequality B
subtract 10.15 both sides
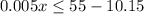
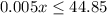
divide by 0.005 both sides
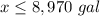
therefore
These customers use at least 4,970 gallons but no more than 8,970 gallons of water per month