64 is a counter example that proves Jim's conjecture as false.
Step-by-step explanation
The number should be less than 100 and need to be both perfect square and perfect cubes.
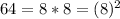
So, 64 is a perfect squared number.
Now,
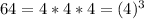
So, 64 is also a perfect cubed number.