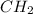Question

Calculate the empirical formula of the hydrocarbon.combustion analysis of a hydrocarbon produced 33.01 g co2 and 9.02 g h2o.

Answer 1

The combustion reaction are as follows.

`C_(x)H_(y) + O_(2) \rightarrow CO_(2) +H_(2)O`

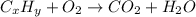
From the given:

Amount of carbon dioxide = 33.01 g

Amount of water = 9.02 g

Let's calculate the number of moles of each compound in the reaction

`Moles of CO_(2) = (Given mass of CO_(2))/(Molar mass of CO_(2))=(33.01 g)/(44.01 g/mol)= 0.75 mole`

`Molesof H_(2)O = (Given massof H_(2)O)/( Molar mass of H_(2)O) = 2 * (9.02 g)/(18 g/ mol) = 1 mole`

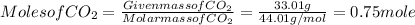
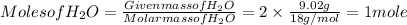
Each value is divided by small value

`Carbon= (0.75)/(0.75) = 1`

`Hydrogen= (1.0)/(0.75) = 1.3 \approx 2`

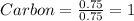
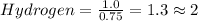
Therefore, Empirical formula of given hydrocarbon is
`CH_(2)`