Question

The perimeter of a rectangle is 64 units. Can the length x of the rectangle can be 20 units when its width y is 11 units?

A.) No, the rectangle cannot have x = 20 and y = 11 because x + y ≠ 64
B.) No, the rectangle cannot have x = 20 and y = 11 because x + y ≠ 32
C.) Yes, the rectangle can have x = 20 and y = 11 because x + y is less than 64
D.) Yes, the rectangle can have x = 20 and y = 11 because x + y is less than 32

Answer 1

Given,
Perimeter of rectangle = 64 units.
Width = y = 11 units


Let,
Length = 20 units, as discussed in the question





Perimeter of rectangle = 2( length + width )


Solving Left Hand Side,


= > Perimeter of rectangle

= > 64 units [ Given ]



Solving Right Hand Side ,


= > 2( 20 + 11 )

= > 2( 31 )

= > 2 × 31


`= > 62`







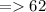
As we can see that 64 ≠ 62

64 ≠ 2( x + y )


Divide by 2 on both sides,


32 ≠ x + y






Hence, option B is correct.