In the figure, we can consider that the base is the side that mesures 12 in and that the height is the side that measures 15 in, since that sides are perpendicular. So, we just need to use the given formula:
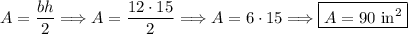
Hence, the area of the triangle is 90 in² (B).