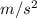a) For the motion of car with uniform velocity we have ,
 , where s is the displacement, u is the initial velocity, t is the time taken a is the acceleration.
, where s is the displacement, u is the initial velocity, t is the time taken a is the acceleration.
In this case s = 520 m, t = 223 seconds, a =0
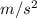
Substituting

The constant velocity of car a = 2.33 m/s
b) We have
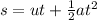
s = 520 m, t = 223 seconds, u =0 m/s
Substituting
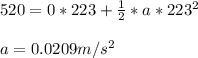
Now we have v = u+at, where v is the final velocity
Substituting
v = 0+0.0209*223 = 4.66 m/s
So final velocity of car b = 4.66 m/s
c) Acceleration = 0.0209