Question

What is the numerical coefficient of the a8b2 term in the expansion of (13a2−4b)6

Answer 1

(13a^2 - 4b)^6 = (13a^2)^6 + 6C1 (13a^2)^5 (-4b)^1 + 6C2 (13a^2)^4 * (-4b)2

The last of the above terms has a^8b^2 as part of it so the required numerical coefficient = 6C2 * 13^4 * (-4)^2

= 15*28561* 16

= 6,854,640 Answer

Answer 2

6854640

Explanation:

Binomial expansion:

`\left(a+b\right)^n=\sum _(r=0)^n\binom{n}{r}a^(\left(n-r\right))b^r`

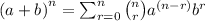
Term where power of second term is r.

`Term=\binom{n}{r}a^(\left(n-r\right))b^r`

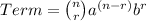
The given expression is

`(13a^2-4b)^6`

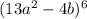
here, n=6.

We need to find the coefficient of the term
`a^8b^2`.

Power of b is 2, so the value of r is 2.

Using binomial expansion, we can find the term
`a^8b^2` with its coefficient.

`=\binom{6}{2}(13a^2)^(\left(6-2\right))(-4b)^2`

`=(6!)/(2!\left(6-2\right)!)\left(13a^2\right)^4\left(-4b\right)^2`

`=15(13)^4(a^2)^4(-4)^2(b)^2`

`=6854640a^8b^2`

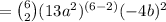
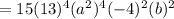
Therefore, the coefficient of the term
`a^8b^2` is 6854640.