Question

The coordinates of the vertices of △RST are R(−3, −1) , S(−1, −1) , and T(−4, −5) .

The coordinates of the vertices of △R′S′T′ are R′(1, −2) , S′(1, 0) , and T′(5, −3) .

What is the sequence of transformations that maps △RST to △R′S′T′?

Drag and drop the answers into the boxes to correctly complete the statement.

A sequence of transformations that maps △RST to △R′S′T′ is a _______followed by a __________.

-reflection across y axis
-translation 1 unit up
-rotation of 180 degress about the orgin
-rotation of 90 degrees counterclockwise about the orgin

Answer 1

A sequence of transformations that maps △RST to △R′S′T′ is a rotation of 90 degrees counterclockwise about the origin followed by a translation 1 unit up.

Explanation:

It is given that the coordinates of the vertices of △RST are R(−3, −1) , S(−1, −1) , and T(−4, −5).

The coordinates of the vertices of △R′S′T′ are R′(1, −2) , S′(1, 0) , and T′(5, −3).

If △RST rotated 90 degrees counterclockwise about the origin, then

`(x,y)\rightarrow (-y,x)`

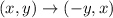
The vertices after rotation are

`R(-3,-1)\rightarrow R_1(1,-3)`

`S(-1,-1)\rightarrow S_1(1,-1)`

`T(-4,-5)\rightarrow T_1(5,-4)`

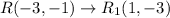
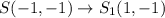
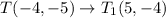
After that if the image translated 1 units up then

`(x,y)\rightarrow (x,y+1)`

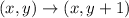
The vertices after rotation followed by translation are

`R_1(1,-3)\rightarrow R'(1,-2)`

`S_1(1,-1)\rightarrow S'(1,0)`

`T_1(5,-4)\rightarrow T'(5,-3)`

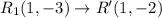
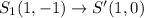
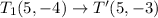
Therefore, a sequence of transformations that maps △RST to △R′S′T′ is a rotation of 90 degrees counterclockwise about the origin followed by a translation 1 unit up.

Answer 2

rotation of 180 degrees about the origin followed by a translation 1 unit up