Answer:
The given point does not lie on the unit circle.
Explanation:
We are given the following information in the question:
A unit circle is a circle with radius of 1 unit and has its center on the origin that is with coordinates (0,0).
Thus, the equation of unit circle is of the form:
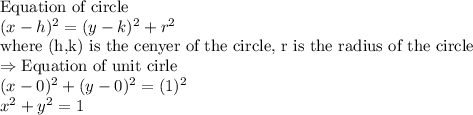
Now, we are given the point
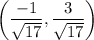
Putting this values in the equation of unit circle, we have:

Thus, the given point does not lie on the unit circle.