Exterior Angle Theorem basically says that if there's an angle that makes a 180° with one of the angles of a triangle, the other two angles of that triangle must be equal to the angle outside.
In the context of this problem, that means m∠SAB is equal to m∠B + m∠C. Putting that into an equation would look like this:
.
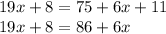From here, we can solve for x with the usual methods. Here's how it would look:
.
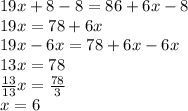With x being six, we can now find the angle measures of all of the angles in the problem by plugging it into the angles that have x and using our rules along with our theorems from there. Let's start with ∠C, which is 6x + 11. It would look like this:
. Now we know that m∠C is 47°, we can find the angle outside through exterior angle theorem again. We can set it up and solve it like this:
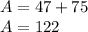
So we know that the exterior angle is 122°. We also know that the interior angle (the one inside that we don't know yet) is a supplementary angle to our exterior angle (meaning that their angles add up to 180° and that they make a straight line). From this, we can find the angle by subtracting 122 from 180. This gets us 58°.
So, your angles measures are the exterior angle being 122°, the interior angle being 58°, and m∠C being 47°. Also, your x value is 6.