Question

Average rate of change

Answer 1

The average rate of change has a relationship with slopes. In this way, we can write the slope of a line as follows:

`Slope=(Change \ y)/(Change \ x)`

To find the Average Rate of Change (ARC), we need to compute the following:

`ARC=(s_(2)-s_(1))/(y_(2)-y_(1))=slope \\ \\ where: \\ \\ s_(2): Number \ of \ students \ in \ 2011 \\ s_(1): Number \ of \ students \ in \ 2001 \\ \\ y_(2): Year \ 2011 \\ y_(1): Year \ 2001`

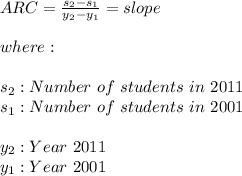
So:

`ARC=(305-775)/(2011-2001)=-47`

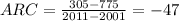
As you can see we have a negative sign. This means that the enrollment at the college has been decreasing at a rate of 47 students per year.