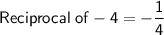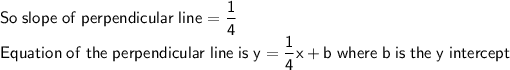Question

Write an equation of the line passing through the point (4,- 3) that is perpendicular to the line 4x+y=3

Answer 1

`\boxed{y = (1)/(4)x -4}`

Explanation:

The slope-intercept form of a line equation is y = mx + b
`\sf\\\\To\;find\;b, \;plug \;in \;the \;values\; of\; (4, - 3) \;which \;corresponds\; to\; x = 4, y = -3 \;into \;the \;above \;equation:\\== > - 3 = (1)/(4)\cdot 4 + b\\\\Switching\;sides,\\(1)/(4)\cdot 4 + b = -3\\\\Simplifying\\1 + b = -3\\\\b = -3 -1 = -4\\So\; the\; equation \;of \;the\; perpendicular\; line \;is: \boxed{y = (1)/(4)x -4}`

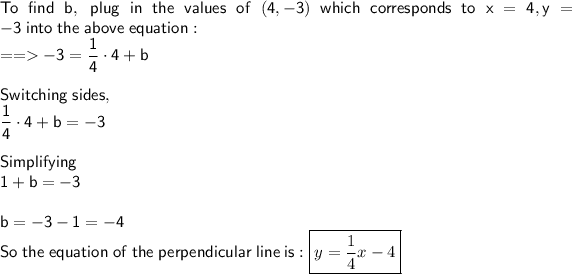
A perpendicular line will have slope is
`-(1)/(m)`

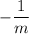
The perpendicular line will have a slope which is the negative of the reciprocal of the first line

Here the line is 4x + y =3

This can be re-written as y = -4x + 3

Slope of this line is -4

`\sf Reciprocal \; of-4 = -(1)/(4)`

`\sf Negative \; of \; reciprocal = -(-(1)/(4)) = (1)/(4)`

`\sf So\;slope\;of\;perpendicular\;line=(1)/(4)\\Equation \;of \;the \;perpendicular\; line\; is \;y = (1)/(4)x + b \;where \;b \;is \;the\; y\; intercept`