the equations are already in slope-intercept form, therefore
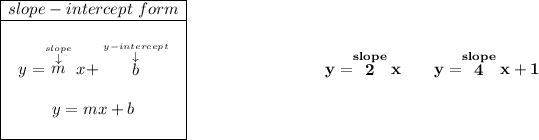
so their slopes are 2 and 4.
parallel lines have exactly the same slope, these ones do not, so they're not parallel.
perpendicular lines, have negative reciprocal slopes, in short, their product gives -1, 2*4 ≠ -1, so they're not perpendicular either.