Question

F(x) 5x/x-2

Identify the vertical and horizontal asymptotes of each rational function.

I need help understanding how to solve problems like this.

Answer 1

Vertical asymptote: x=2

Horizontal asymptote: y=5

Explanation:

Hi there!

The parent function for a rational function has a horizontal asymptote at y=0 and a vertical asymptote at x=0.

First, we want to perform long division on 5x/x-2. Please check the image attached.

The result is
`5+\displaystyle(10)/(x-2)`.

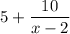Here are the transformations of a rational function:

`f(x)=\displaystyle(a)/(b(x-h))+k`

a = vertical stretch

b = horizontal stretch

h = horizontal translation

k = vertical translation

Stretches don't change the location of the asymptotes, only the translations do.

According to
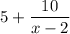`5+\displaystyle(10)/(x-2)`, we applied a horizontal translation of 2 units right and a vertical translation of 5 units up. Therefore, our asymptotes are now x=2 and y=5.

I hope this helps!