Question

Simplify the rational expression. State any restrictions on the variable.

Answer 1

we are given

`((n^4-10n^2+24))/((n^4-9n^2+18))`

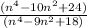
Firstly, we will factor numerators and denominators

`((n^4-10n^2+24))/((n^4-9n^2+18))=((n^2-6)(n^2-4))/((n^2-3)(n^2-6))`

we can see that

n^2 -6 is factor on both numerator and denominator

so, it will get cancelled

and n^2 -6 can not be equal to 0

so, one of restriction is

`n^2-6\\eq 0`

`n\\eq -+ √(6)`

we can simplify it

`((n^4-10n^2+24))/((n^4-9n^2+18))=((n^2-4))/((n^2-3))`

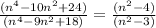
we know that denominator can not be zero

`n^2-3\\eq 0`

`n\\eq -+ √(3)`

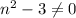
so, option-B.......Answer