The inverse function, denoted
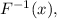 of a one-to-one function
of a one-to-one function
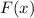 is defined as
is defined as
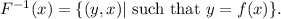
Main properties:
1.
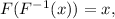 x in the domain of
x in the domain of
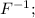
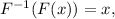 x in the domain of F.
x in the domain of F.
2. The domain of F is equal to the range of
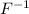 and the range of F is equal to the domain of
and the range of F is equal to the domain of
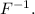
From these properties you can state that options B, C, D and E are true and points A and F are false.