Question

Write an equation in point-slope form for the line.
(1,3)
(0,1)
m=4

Answer 1

Given coordinates (1,3) and (0,1).

In order to find the equation of line, we need to find the slope between given coordinates.

We know slope formula,

m=
`(y2-y1)/(x2-x1)`

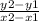
We have (x1,y1) = (1,3) and (x2,y2) = (0,1).

Plugging values of x1,y1,x2 and y2 in slope formula, we get

`m=(1-3)/(0-1)`

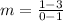
Simplifying top and bottom of the fraction we got for slope

`m=(-2)/(-1)=2`

We got slope m=2.

We are given second point (0,1), where x-coordinate is 0 and y-coordinate is 1.

y-intercept is a point on y-axis, where x=0.

Therefore, y-intercept = 1.

Now, applying slope-intercept form of the linear equation

y=mx+b, where m is the slope and b is y-intercept.

m=2 and b=1.

Plugging values of m and b in slope-intercept form, we get

y=2x+1.

Therefore, point-slope form for the line is y=2x+1.