Question

Find the perimeter p of ▱jklm with vertices j(2,2), k(5,3), l(5,−3), and m(2,−4). Round your answer to the nearest tenth, if necessary.

Answer 1

Consider the vertices of parallelogram JKLM with vertices J(2,2) , K(5,3) , L(5,-3) and M(2,-4).

Perimeter JKLM = Length JK + Length KL + Length LM + Length JM

Length JK = (2,2) (5,3)

The length(or distance) between two points say
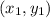`(x_(1),y_(1))` and
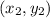`(x_(2),y_(2))` is given by the distance formula:

`\sqrt{(x_(2)-x_(1))^(2)+(y_(2)-y_(1))^(2)}`

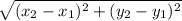
Now, length JK =
`\sqrt{(5-2)^(2)+(3-2)^(2)}`

=
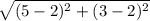
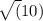`\sqrt(10)` units

Since, JKLM is a parallelogram. In parallelogram opposite sides are equal in length.

Therefore, LM =
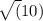`\sqrt(10)` units

Now, length KL =
`\sqrt{(5-5)^(2)+(-3-3)^(2)}`

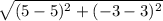
= 6 units

Since, JKLM is a parallelogram. In parallelogram opposite sides are equal in length.

Therefore, JM = 6 units

Perimeter of JKLM =
`\sqrt(10)` +
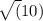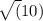`\sqrt(10)` + 6 + 6

= 2
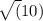`\sqrt(10)` + 12

= 18.324

Rounding to the nearest tenth, we get

= 18.3 units.

Therefore, the perimeter of JKLM is 18.3 units.