Question

How many grams of sodium phosphate monobasic would we add to a liter and how many grams of sodium phosphate dibasic would we add to a liter to make a 0.1 m phosphate buffer at ph 6.86?

Answer 1

The correct answer for Mass of Na₂HPO₄ = 4.457 g and mass of NaH₂PO₄ = 8.23 g

Given : pH = 6.86

Total concentration of Phosphate buffer = 0.1 M

Asked : Mass of Sodium phosphate monobasic (NaH₂PO₄) = ?

Mass of Sodium phosphate dibasic(Na₂HPO₄)= ?

Following steps can be done to find the masses of NaH₂PO₄ and Na₂HPO₄ :

(In phosphate buffer , Na+ ion from NaH₂PO₄ and Na₂HPO₄ acts as spectator ion , so only H₂PO₄⁻ and HPO₄²⁻ will be considered )

Step 1 : To find pka

H₂PO₄⁻ <=> HPO₄²⁻

The above reaction has pka = 7.2 ( from image shown )

Step 2 : Plug values in Hasselbalch- Henderson equation .

Hasselbalch -Henderson equation is to find pH for buffer solution which is as follows :

`pH = pka + log([A^-])/([HA])`

pH = 6.86 pKa = 7.2

`6.86 = 7.2 + log ([HPO_4^2^-])/([H_2PO_4^-])`

Subtracting both side by 7.2

`6.86-7.2 = 7.2 -7.2+ log ([HPO_4^2^-])/([H_2PO_4^-])`

`-0.34 =  log ([HPO_4^2^-])/([H_2PO_4^-])`

Removing log

`10^-^0^.^3^4 =   ([HPO_4^2^-])/([H_2PO_4^-])`

`([HPO_4^2^-])/([ H_2PO_4^-]) = 0.457` ---------------- equation (1)

Step 3 : To find molarity of H₂PO₄⁻ and HPO₄²⁻

Total concentration of buffer = [H₂PO₄⁻] + [HPO₄²⁻] = 0.1 M

Hence, [H₂PO₄⁻ ] + [ HPO₄²⁻ ] = 0.1 M

Assume [H₂PO₄⁻ ] = x

So , [x ] + [ HPO₄²⁻ ] = 0.1 M

[ HPO₄²⁻ ] = 0.1 - x

Step 4 : Plugging value of [H₂PO₄⁻ ] and [ HPO₄²⁻ ]

[H₂PO₄⁻ ] = x

[ HPO₄²⁻ ] = 0.1 - x

Equation (1) = >
`([HPO_4^2^-])/([ H_2PO_4^-]) = 0.457`

Plug value of [H₂PO₄⁻ ] and [ HPO₄²⁻ ] ( from step 3 ) into equation (1) as :

`([0.1 - x ])/([ x]) = 0.457`

Cross multiplying

`0.1 - x  = 0.457 x`

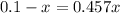
Adding x on both side

`0.1 -x + x = 0.457 x + x`

`0.1  = 1.457 x`

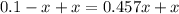
Dividing both side by 1.457

`(0.1)/(1.457) = (1.457 x )/(1.457)`

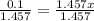
x = 0.0686 M

Hence , [H₂PO₄⁻ ] = x = 0.0686 M

[ HPO₄²⁻ ] = 0.1 - x

[ HPO₄²⁻ ] = 0.1 - 0.0686

[ HPO₄²⁻ ] = 0.0314 M

Step 5 : To find moles of H₂PO₄⁻ ( NaH₂PO₄) and HPO₄²⁻ (Na₂HPO₄ ) .

Molarity is defined as mole of solute per 1 L volume of solution .

Molarity of NaH₂PO₄ = 0.0686 M or 0.0686 mole per 1 L

Molarity of Na₂HPO₄ = 0.0314 M or 0.0314 mole per 1 L

Since that volume of buffer solution is 1 L , so Molarity = mole

Hence Mole of NaH₂PO₄ = 0.0686 mol

Mole of Na₂HPO₄ = 0.0314 mol

Step 6 : To find mass of Na₂HPO₄ and NaH₂PO₄

Moles of Na₂HPO₄ and NaH₂PO₄ can be converted to their masses using molar mass as follows :

Molar mass of Na₂HPO₄ =
`141.96 (g)/(mol)`

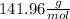
Molar mass of NaH₂PO₄ =
`119.98 (g)/(mol)`

`Mass (g) = mole (mol)* molar mass((g)/(mol))`

`Mass of Na_2HPO_4 = 0.0314 mol * 141.96 (g)/(mol)`

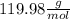
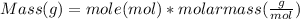
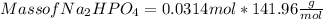
Mass of Na₂HPO₄ = 4.457 g

`Mass of NaH_2PO_4 = 0.0686 mol * 119.98 (g)/(mol)`

Mass of NaH₂PO₄ = 8.23 g