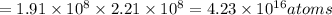Question

Using arrangement (a), how many rb atoms could be placed on a square surface that is 9.5 cm on a side? the diameter of a rubidium atom is 4.95å. we will consider two different ways of placing the atoms on a surface. in arrangement (a), all the atoms are lined up with one another. arrangement (b) is called a close-packed arrangement because the atoms sit in the "depressions" formed by the previous row of atoms:

Answer 1

Part a

Answer:
`3.64*10^(16)`

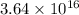
For the arrangement considered in part a, all the atoms are aligned side by side along the side of the square surface.

Along one side of the square, the number of atoms that could fit
`=\frac {length\hspace{1mm}of\hspace{1mm}side}{diameter\hspace{1mm}of\hspace{1mm}atom}=(9.5*10^(-2)m)/(4.95*10^(-10)m)=1.91*10^8 atoms`

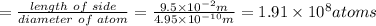
This is along one side. On the entire surface:

Number of atoms
`= (1.91*10^8 )^2=3.64*10^(16) atoms`

Part b

Answer:
`4.23*10^(16)`

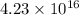
consider the attached figure below:

The next layer of atoms are filled in the depressions of the first layer. The vertical distance between the two atoms would change. Consider an equilateral triangle drawn by joining the centers of the three atoms.

The atoms along the horizontal side would be aligned side by side; same as above
`=1.91*10^8 atoms`

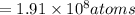
The number of atoms along vertical side would vary. vertical distance between two atoms can be calculate using equilateral triangle as shown below.

an equilateral triangle has all the angles
`= 60^o`

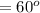
Let the vertical distance be y.

Then,
`y = 2r sin60^o`

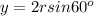
where r is the radius of each atom.
`2r= 4.95*10^(-10) m`

`y=4.95*10^(-10) m* sin60^o=4.28*10^(-10) m`

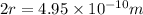
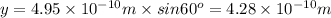
The number of atoms along the vertical side
`= (9.5*10^(-2)m)/(4.28*10^(-10)m)=2.21*10^8 atoms`

Total number of atoms in this kind of arrangement
`= 1.91*10^8*2.21*10^8=4.23*10^(16) atoms`