Answer:
The type of transformation is the reflection across the x-axis.
Explanation:
We are given a parent function f(x) by:
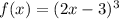
and the transformed function g(x) is given by:
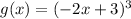
which could also be written as:
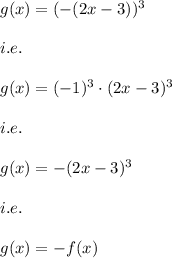
i.e. the transformation g(x) is obtained by reflecting the parent function f(x) across the x-axis.
( Since, when the reflection of a function is done across the x-axis then the x-coordinate of the point of the function remains the same and the y-coordinate of the point takes the negative sign.
i.e. f(x) → -f(x)
i.e.
(x,y) → (x,-y) )