Question

Find the zeros of g(x) = -x^2 + 6x - 8

Answer 1

(Remember to set g(x) to zero).

For this, I will be completing the square. Firstly, what two terms have a product of 8x^2 and a sum of 6x? That would be 4x and 2x. Replace 6x with 2x + 4x:
`0=-x^2+2x+4x-8`

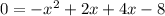
Next, factor -x^2 + 2x and 4x - 8 separately. Make sure that they have the same quantity on the inside of the parentheses:
`0=-x(x-2)+4(x-2)`

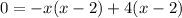
Next, you can rewrite the equation as
`0=(-x+4)(x-2)`

Now apply the Zero Product Property:

`-x+4=0\\-x=-4\\x=4\\\\x-2=0\\x=2`

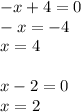
The zeros of this equation are 4 and 2.