Question

Tickets to a basketball game were $2.50 each for students and $4.25 each for adults. if a total of 183 tickets were sold and $538 was collected from the ticket sales, then how many of each type of ticket were sold?

Answer 1

Let's establish our unknown numbers with the variables x and y:

x amount of students
y amount of parents


We can establish these equasions:

x + y = 183 (number of students + number of parents)
2.50x + 4.25y = 538 (the amount each group paid)


To make things easier, we can put x or y from the first equasion on to the right side of that same equasion. I switched x in my case

`x + y = 183 \\ y = 183 - x`
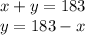
We insert that into our second equasion and solve it

`2.50x + 4.25(183 - x) = 538 \\ 2.50x + 777.75 - 4.25x = 538 \\ 2.50x - 4.25x = 538 - 777.75 \\ - 1.75x = - 239.75 \\ x = ( - 239.75)/( - 1.75) \\ x = 137`
We now know that x = 137. If the total is 183, we just have to subtract 137 from that number to find out the value of y

`183 - 137 = 46`
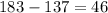
RESULT:
x = 137 ==> 137 student tickets sold
y = 46 ==> 46 adult tickets sold