These are the steps to compute the skew:
- Compute the average of the dataset: sum all of the elements and divide by how many they are:
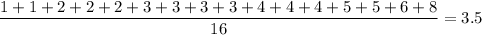
- Compute the standard deviation: subtract the average from all elements, square the result, compute the average of this new dataset and take the square root of the result:
When you subtract the mean, you have
-2.5, -2.5, -1.5, -1.5, -1.5, -0.5, -0.5, -0.5, -0.5, 0.5, 0.5, 0.5, 1.5, 1.5, 2.5, 4.5
When you square the results, you have
6.25, 6.25, 2.25, 2.25, 2.25, 0.25, 0.25, 0.25, 0.25, 0.25, 0.25, 0.25, 2.25, 2.25, 6.25, 20.25
The average of this new dataset is
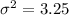
Whose square root, i.e. the standard deviation, is
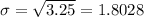
- Return to the dataset where you subtracted the mean from each point, and divide each point by the standard deviation:
-1.38675, -1.38675, -0.83205, -0.83205, -0.83205, -0.27735, -0.27735, -0.27735, -0.27735, 0.27735, 0.27735, 0.27735, 0.83205, 0.83205, 1.38675, 2.49615
-2.666828, -2.666828, -0.576035, -0.576035, -0.576035, -0.021335, -0.021335, -0.021335, -0.021335, 0.021335, 0.021335, 0.021335, 0.576035, 0.576035, 2.666828, 15.552940
- Sum all these numbers together: the result is 12.289.
- Divide this number by the total number of elements in the dataset:
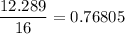
And this is the skew.