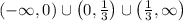Question

Need some help with my last problem for the night.

Determine the domain of tbe function (f o g)(x) where f(x) = 3x-1/x-4 and g(x) = x+1/x

Answer 1

We are given

f(x)=
`(3x-1)/(x-4)`

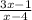
g(x)=
`(x+1)/(x)`

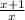
\to find f o g, replace all the x in f(x) with g(x)

`f o g = (3((x+1)/(x))-1)/(((x+1)/(x))-4)`

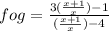
= Simplify by taking least common denominator and reciprocal.

Taking the least common denominator, we get

`(((2x+3)/(x)))/(((-3x+1)/(x)))`

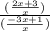
Taking reciprocal, we get

`(2x+3)/(x)\ast (x)/(-3x+1)`

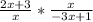
Simplifying the expression, we get

`f o g =(2x+3)/(-3x+1)`

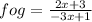
To find the domain of a composite function, we need to find the domain of f o g and the first function i.e g(x)

To find the domain of f o g, equate the denominator to 0

`-3x+1=0`

`x=(1)/(3)`

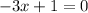
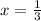
It means, domain is all real numbers except
`x=(1)/(3)`

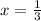
Domain of g(x) is all real numbers except
`x=0`

combined domain is
`\left ( -\infty ,0 \right )\cup \left ( 0,(1)/(3) \right )\cup \left (  (1)/(3),\infty \right )`