Question

consider the expressions ab^2 and a^2 b^2 . are the two expressions equivalent? which properties did you use to prove or disprove the equivalency

Answer 1

Yes,distributive property

Answer 2

Answer: No, they are not equivalent.

Explanation:

Since we have given that

`ab^2\ and\ a^2b^2`

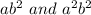
No, the two expressions are not equivalent.

As we know that

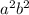`a^2b^2` can be written as
`(ab)^2`

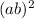
but
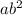`ab^2` can't be written as that .

`x=ab^2\\\\y=a^2b^2\\\\`

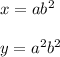
Suppose if they are equivalent then,

then,
`x=y\\\\frac{x}{y}=1`

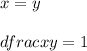
So, if we put the values of x and y we get that

`(x)/(y)=(ab^2)/(a^2b^2)=(1)/(a)\\eq 1`

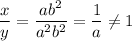
Hence, they are not equivalent.