Question

A ball is thrown upward from the top of a building. the function below shows the height of the ball in relation to sea level, ft, in feet, at different times t in seconds: f(t) = -16t2 + 48t + 100 the average rate of change of f(t) from t = 3 seconds to t = 5 seconds is ___ feet per second.

Answer 1

80 ft/s upward

Explanation:

The average rate of change can be determined by calcualting the height of the ball at the initial time t=3 and final time t=5. We can substitute these values in the expression:

t=3

`=-16\cdot{3^2}+48\cdot{3}+100=-144+144+100=100`

t=5

`=-16\cdot{5^2}+48\cdot{5}+100=-400+240+100=-60`

We can calculate the average change of rate by calculting the gradient of a straight line with the points (3, 100) and (5,-60):

`m=(y_2-y_1)/(x_2-x_1)=(-60-100)/(5-3)=-80`

Answer 2

average rate of change is the slope (of the line) between the two coordinates

f(t) = -16t² + 48t + 100

f(3) = -16(3)² + 48(3) + 100 = -144 + 144 + 100 = 100 (3, 100)

f(5) = -16(5)² + 48(5) + 100 = -400 + 240 + 100 = -60 (5, -60)

m =
`(y2 - y1)/(x2 - x1)` =
`(100 - (-60))/(3 - 5)` =
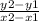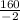`(160)/(-2)` = -80

Answer: -80 ft/sec